Qualifying teammate battles seem to be of great interest to the current F1 community. While I’m a firm believer that Sundays are much more important than Saturdays, I will accept that qualifying has a great impact on a race, especially when you consider how hard it is to overtake with the latest generation F1 cars.

Analysis

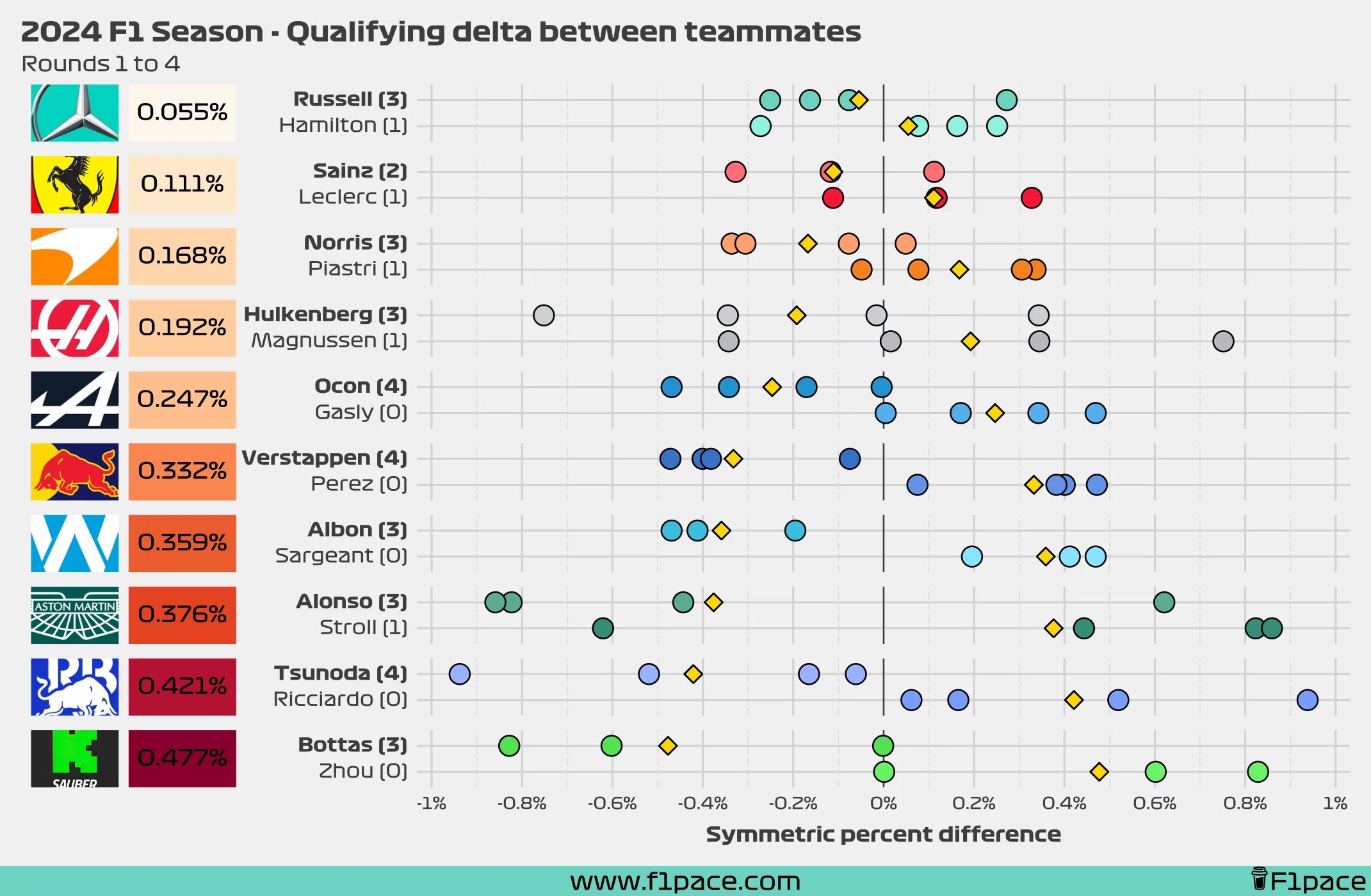
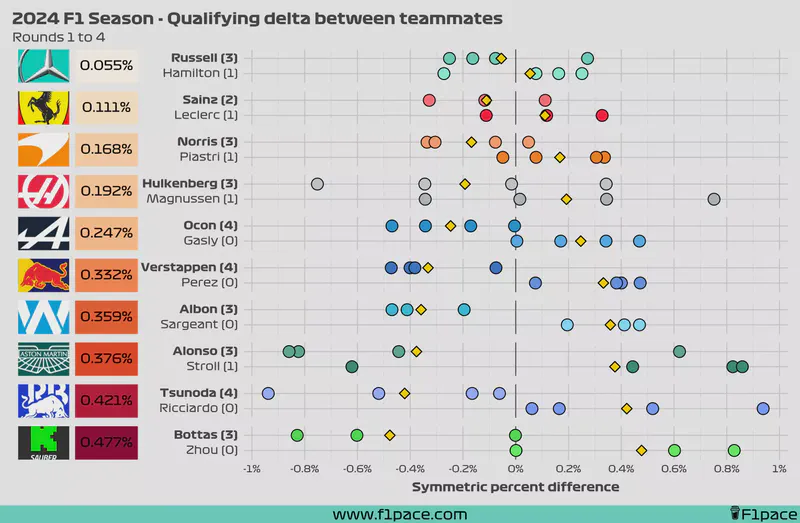
After the first 4 races of the 2024 F1 Season, the smallest delta between teammates is now found at Mercedes with an average delta of just 0.055%. The largest delta has changed too and is now seen at the Sauber F1 team, with Valtteri Bottas beating Zhou Guanyu in each of the previous 3 races, with an average margin of 0.477%.

On a personal note, I found it quite interesting to see that the difference between Alex Albon and Logan Sargeant is 0.359%, which ranks 7th in terms of magnitude compared to the rest of the teams. Currently, Lance Stroll, Daniel Ricciardo and Zhou Guanyu are getting beaten by a larger margin by their respective teammates than Logan by Albon at Williams.

Methodology

I calculated the delta between teammates by using the symmetrical percent difference. To find out why I did this, check the “issues” section after the analysis.

For each race and for the highest qualifying session that both drivers from the same team reached I calculated the symmetric percent difference. Negative values mean that a driver was faster than his teammate, while positive values mean that the driver was slower than his teammate. A difference of 0% means that both drivers were just as fast.

I calculated the values for each race for each team and plotted them as individual data points in the chart. I then calculated the average of these values for the season (so far) and displayed it the left side of the plot, next to the team logo. Smaller overall values represent that both teammates were more evenly matched during quali, while larger overall values show a greater gap between teammates.

Additionally, on the left-hand side of the chart next to the driver’s name, I also added the number of times a particular driver has been faster than his teammate in quali.

Finally, I added a gold-coloured diamond to show the average gap between teammates. This number will be equal to the overall value displayed on the left side of the plot, next to the team logo.

Issues!

One of the main issues when gathering data from multiple races is that the deltas will change depending on the length of each track. A delta of 0.1 seconds in a short track (say, 1:05 per lap) will be greater than a delta of 0.1 seconds in a long track such as Spa (~ 1:45).

One way we can standardize the data is by converting the deltas to percentages, but there is one big issue with this. The traditional way of calculating a percent difference is with the following formula:

$$ Percent\ difference = 100\times\frac{value1-value2}{value2} $$

The main problem is that this value is not symmetrical. This means that if I reverse the order of value 1 and value 2, the final percent difference will be different.

$$ Percent\ difference = 100\times\frac{80-90}{90}=-11.11\% $$ $$ Percent\ difference = 100\times\frac{90-80}{80}=12.5\% $$

You can see that the percentages are not reversible, even though in both cases we’ve changed the original value by 10 units.

One way we can solve this problem is by using the symmetric percent difference, which is calculated by using the following formula:

$$ Symmetric\ percent\ difference = 100\times\frac{value1-value2}{(value1+value2)/2} $$ This formula is reversible, meaning that regardless of the order of the values, we will get the same result. Because of this, I decided to use the symmetric percent difference formula as the basis for the analysis.